SSH Encryption

Secure Shell (SSH) is a cryptographic network protocol meant to secure communications over an insecure connection between network devices. One of the ways SSH does this is by using a hybrid approach between asymmetric public/private key- and symmetric cryptography.

SSH is most commonly used as a means for secure remote login and command execution, often in the context of a client-server interaction, but is also often used for authentication and in file transfers protocols (SFTP / SCP).

This article will discuss and explore, among other things, the possible ways of creating SSH-keys, the underlying methods of encryption and some general best practices concerning interactions with servers and ssh key management. It is therefore complementary to the article: "SSH for beginners."

Introduction

A cryptographic system like SSH is used when communications must be withheld from third parties (confidentiality), the identity of the other party needs to be verified (authenticity) and/or when you want to make sure that received messages have not been altered (integrity). To understand how communications are encrypted in SSH we first need to understand some basic terms and concepts. The difference between asymmetric and symmetric cryptography is a good place to start.

Symmetric vs Asymmetric

Symmetric cryptography is something probably most people are and have been familiar since their youth. An example: The alphabet has 26 characters and we assign each position a number (a='1', b='2' etc.), then we proceed to "shift" or "rotate" each character for n steps down this sequence. If we take n=1 for example, so that each letter gets "bumped up" one value and effectively taking the place of the character that was there before. This gives: z='1', a='2', b='3' etc). This algorithm is referred to as ROTn (or Caesar Cipher) where n would be the number of steps to rotate the characters. N would simultaneously be both the key to encrypt and to decrypt the message (hence the name 'symmetric'). An example message would be "Hello World", which we would encrypt with ROT13 to "Uryyb Jbeyq" and could decrypt with the same key back to "Hello World". Many people have experimented with this algorithm to encrypt messages during childhood and almost all people discover quite quickly how easy it is to break such encryption. Of course many more complicated versions exist which are not as easily solved by hand but suffer the same underlying weaknesses as this simplified algorithm does and can get cracked quite easily with modern computing power using methods like frequency analysis. The stress here is on keeping the key a secret, available only to the trusted parties and this is where the distinction with asymmetrical cryptography comes into play.

Asymmetric cryptography, using different keys to encrypt and decrypt, still works very similarly to what we have discussed before. There is an encryption algorithm available (previously the ROTn algorithm but in next parts we will discuss others like RSA and Ed25519) which "jumble" up the message depending on the specific key that is provided (this key will be known as the "public key" from now on) but where it differs is the decryption. Technically speaking the public key used to encrypt still contains all of the information needed to decrypt the message but this information is obfuscated by complexity and a modern computer's inability to retrieve this information in an acceptable amount of time. The other key in the pair (known as the "private key" from now on) is created alongside with the public key and allows for quick decryption of the message. This difference in decryption speed allows for safe sharing of public keys so that anyone wanting to send private data can encrypt it but only the holder of the private key can decrypt easily. The analogy that often comes up is that of the padlock and key: anyone who is provided a padlock (public key) can lock a message in a box, but only the person with the key (private key) is able to open the box and read the message.

Mathematical Concepts

To fully grasp the mathematical complexities of modern-day cryptographic systems one has to delve deep into number theory. This however, falls outside of the scope of this article and we will therefore only superficially touch on these subjects. Two concepts needed to start understanding these are modulo and primes.

When two integers are divided we obtain a quotient (e.g. how often the integer fits in the other) and a remainder. For example 19/5 = 3 with remainder 4, the remainder is referred to as the modulo. In different notation this becomes: 19mod5 = 4.

Prime numbers are positive integers which are only divisible by 1 and themselves (leaving 0 as remainder). The unique-prime-factorization theorem states that any positive integer greater than 1 can be written as a unique product of prime numbers. These are important ideas to keep in mind when moving on to the next part where we will discuss the inner workings of the RSA-algorithm.

RSA vs Elliptic Curve

RSA

A message can be encrypted into a cipher text (C) if we first represent it as a number (M) according to a character encoding standard like ASCII. Here each character is represented using 7 bits for a total of 128 unique characters, although other encoding standards are available (UTF-8 / -16, extended ASCII etc.) which use more bits per character and thus support a greater variety of characters. "Hello World" would turn into the following sequence of numbers: "072 101 108 108 111 032 087 111 114 108 100". The RSA algorithm proceeds as follows:

   1) Create a numerical representation of original message (similar to our "Hello World"-example)
   2) Choose two (very large "random") primes p and q, which result into: n = p * q
   3) Choose a number d which is a relative prime of: (p-1) * (q-1).
      By relative prime is meant that the greatest divisor of d and (p-1) * (q-1) must equal '1'. 
      d can be chosen relatively easily by picking a prime number larger than either p or q
   4) Compute a number e using numbers d / p / q and Euclid's Algorithm. 

RSA encryption / decryption

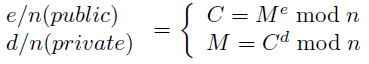
e and n are provided as the public key and are meant to encrypt. d and n are kept as the private key and are meant for decryption. The two formulas presented are used to gain the cipher text from a desired message (encryption) or the message from the received cipher text (decryption). A method to gain the value of M^e / M^d is given by the original authors of the RSA-algorithm by "exponentiation by repeated squaring and multiplication". They refer to volume 2 of Donald Knuth's algorithm bible "The Art of Programming" for more efficient approaches. For an introduction some good materials are also available through the Khan Academy: "Modular Exponentiation".

Example

   1) We choose a prime p = 5  and a prime q = 11. The product of these two result in n = p * q = 5 * 11 = 55.
   2) t = (p-1) * (q-1) = 4 * 10 = 40. This gives us some options to choose a value d, in this case we choose d = 23.

     At this moment we know almost every number needed for both encryption and decryption of our secret message. 
     Only e has still to be computed. This is done using Euclid's Extended Greatest Common Divisor-algorithm.
     e's relation with d is described as such: e * d mod n = 1.

See Also

• The original papers on public-private key based cryptography and the RSA algorithm provide an essential starting point for further reading on the topic: "RSA" / "New directions in Cryptography - by W. Diffie and E. Hellman"